Random Fact #4
Today’s Fact: Foot On Water
Andi came up with an insane question: How wide does a human foot have to be for a human to stand on water? Of course, he came up with something insane, reproduced here.
Foot On Water, Andi Gu
Relevant Parameters
Let \(m\) be the mass of some person, let \(R\) be the radius of his foot (which we model as a circle), and let \(\gamma\) be the surface energy of water (i.e. surface tension).
We make a simplistic model in which the water deforms in a circularly symmetric pattern around the foot, that when viewed at a cross-section appears as follows:
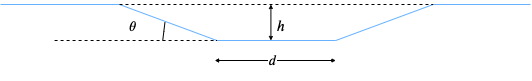
Derivation of Foot Size
The increase in the water surface area is
\[\begin{align*} \Delta A &= \int_{R}^{R+\frac{h}{\tan \theta}} 2\pi r (\frac{1}{\cos \theta}-1) d{r} \\ &= \pi (\frac{h^2}{\tan^2 \theta} + \frac{2Rh}{\tan \theta}) \frac{1-\cos \theta}{\cos \theta} \end{align*}\]Since \(\Delta E=\gamma \Delta A\), we have:
\[\begin{align*} F_{up} &= \frac{\partial E}{\partial h} \\ &= \gamma \pi (\frac{1-\cos \theta}{\cos \theta}) (\frac{2h}{\tan^2 \theta}+\frac{2R}{\tan \theta}) \\ &= 2\gamma\pi (\frac{1-\cos \theta}{\cos \theta}) (\frac{h+R \tan \theta}{\tan^2 \theta}) \\ &\approx 2\gamma \pi R \frac{1-\cos \theta}{\sin \theta} \end{align*}\]with the last step following since it is reasonable to assume \(R \gg h\)
The only remaining variable is \(\theta\) – it is reasonable to assume a small angle \(\theta\) (i.e. \(\theta \approx \frac{\pi}{10}\), so that this adds a factor of approximately 0.1). With \(\gamma=72.8\) dynes per centimeter and \(m=80\) kilograms, \(R \approx 13 \text{km}\).
